Zero Perturbation

This shows a Zero Constellation for a Binary MOXZ signal with K=6 Conjugate-reciprocal zero pairs on circles (black) with radii R=1.225 and 1/R=0.816 under an additive noise perturbation. The more red the color, the higher the noise power. Numerating the bit order from the positive real-axis counter-clockwise, this transmit constellation corresponds to the data-message [1 0 0 0 0 0]. The right picture shows the same 6 transmit zeros observed in a random channel of length L=4, resulting in 3 (red) channel zeros. In this case, the DiZeT (Direct Zero Testing) decoder or a root finding decoder would decode the transmit message error-free.

Physical Layer Security

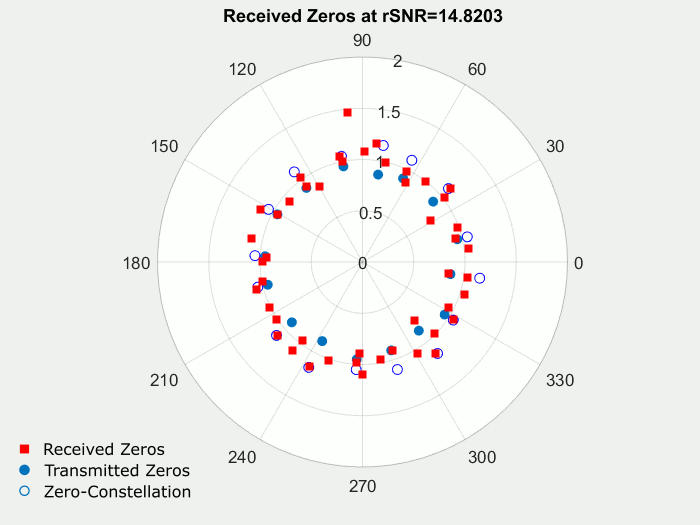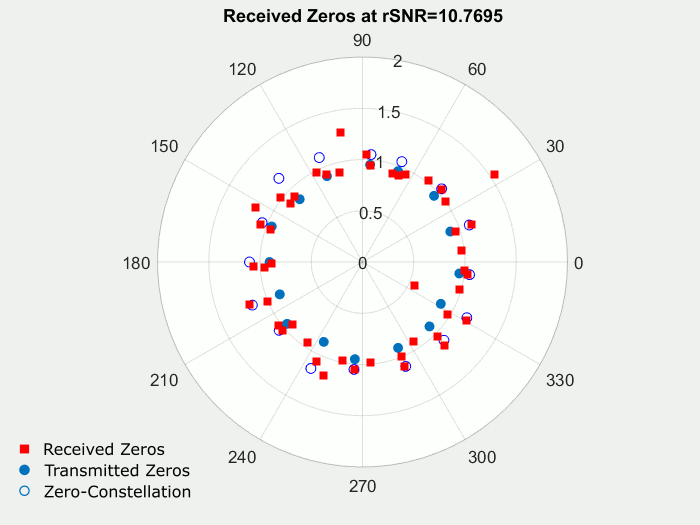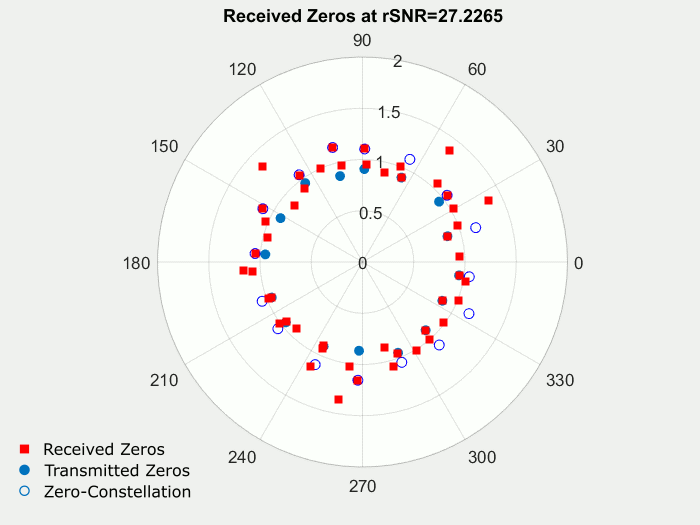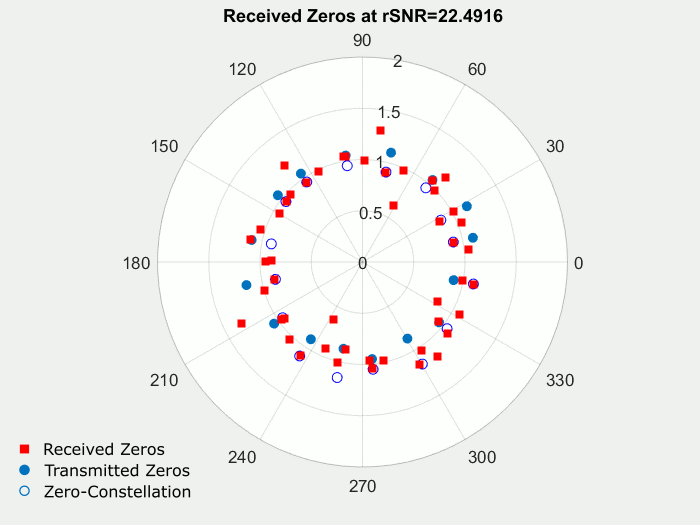
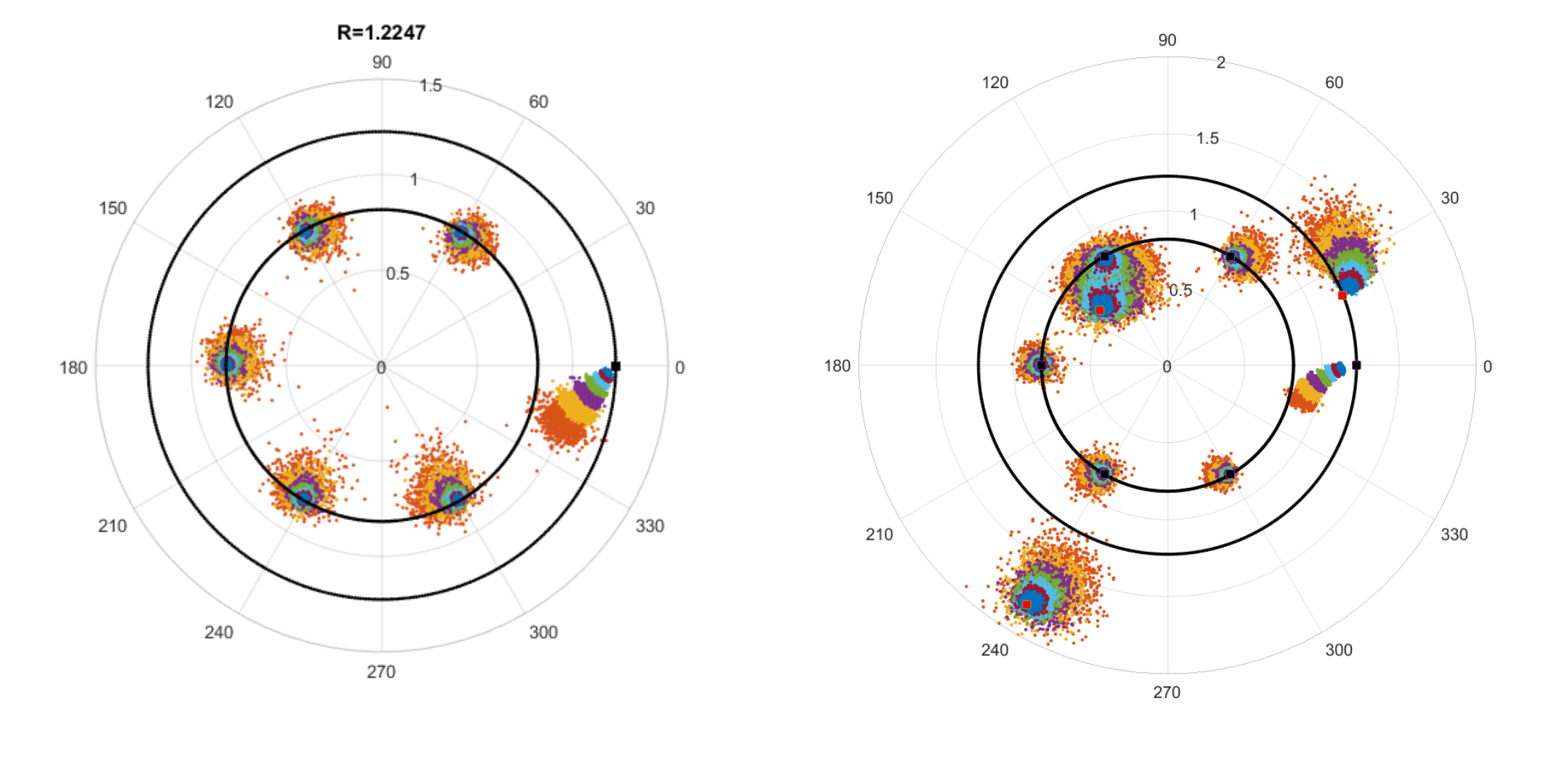
The animation shows the zeros of random BMOXZ signals for K=33 conjugated-reciprocal zero-pairs (blue circles). The actual transmitted zeros are the filled blue circles. The red squares are the received noisy zeros in the presence of an i.i.d Channel impulse response of length L=16, generating 15 random channel zeros. In this case, we changed the zero-constellations by phase and radii, such that it looks like a random zero-codebook, which makes it harder to identify the constellation/modulation scheme. The constellation alternation can be seen as a secret analog physical layer code. Without this code it is impossible to identify the data zero neither it is possible to separate the data zeros from the channel, which gives BMOXZ an intrinsic physical layer security.